Procter and Gamble on rise?

According to [1], the model for Procter and Gamble (PG) is defined by the index of food away from home (SEFV - CUUS0000SEFV) and that of rent of primary residency (RPR). The former CPI component leads the share price by 3 months and the latter one leads by 8 months. Figure 1 depicts the overall evolution of both involved indices. These two defining components provide the best fit model between August 2009 and June 2010. Relevant coefficients are negative and positive, respectively. The slope of time trend is also positive.

So, the best-fit 2-C model for PG(t) is as follows:

PG(t) = -5.88SEFV(t-3) + 3.43RPR(t-8) + 17.60(t-2000) + 174.08

where t is calendar time.

The predicted curve in Figure 2 leads the observed price by 4 months with the residual error of $2.08 for the period between July 2003 and June 2010. In other words, the price of a PG share is completely defined by the behaviour of the two CPI components.

The model does predict the share price in the past and foresees a period of modest growth in the near future. This contradicts the predicted overall fall in the S&P 500 in 2010. One might expect a slight growth in PG share price, but this deviation also can manifest the end of the period where the model is valid. This deviation may be induced by the change in the trend in both or one of the underlying CPIs: SEFVand RPR, as Figure 1 illustrates.

Figure 1. Evolution of the price of SEFV and RPR.

Figure 2. Observed and predicted PG share prices. The original prediction, i.e. the prediction three months before actual time, is shown by red line. Black diamonds present the original line shifted 3 months ahead to fit actual data.

Figure 3. Residual error of the model. Mean residual error is 0 with standard deviation of $2.08. The largest errors were observed in 2007.

References

Kitov, I. (2010). Deterministic mechanics of pricing. Saarbrucken, Germany, LAP Lambert Academic Publishing.

PepsiCo share price

According to [1], the model for PepsiCo (PEP) is defined by the index of food at home (FH) and that of information technology (IT). The former CPI component leads the share price by 4 months and the latter one leads by 8 months. Figure 1 depicts the overall evolution of both involved indices. These two defining components provide the best fit model between August 2009 and June 2010. Both coefficients are negative and the slope of time trend is positive.

So, the best-fit 2-C model for PEP(t) is as follows:

PEP(t) = -1.41FH(t-12) – 8.54IT(t-4) +1.54(t-2000) + 416.80

where t is calendar time.

The predicted curve in Figure 2 leads the observed price by 4 months with the residual error of $2.26 for the period between July 2003 and June 2010. In other words, the price of a PEP share is completely defined by the behaviour of the two CPI components.

The model does predict the share price in the past and foresee no change in the near future. This is one of rare shares that is not predicted to drop with the overall fall in the S&P 500 in 2010.

 

Figure 1. Evolution of the price of FH and IT.

Figure 2. Observed and predicted PEP share prices. The original prediction, i.e. the prediction four months before actual time, is shown by red line. Black diamonds present the original line shifted 4 months ahead to fit actual data.

Figure 3. Residual error of the model. Mean residual error is 0 with standard deviation of $2.26. The largest errors were observed in 2008.

References

Kitov, I. (2010). Deterministic mechanics of pricing. Saarbrucken, Germany, LAP Lambert Academic Publishing.

IBM share price on decline

According to [1], the model for IBM (IBM) is defined by the index of motor vehicle maintenance and repair (MVR - CUUR0000SETD) and that of transportation services (TS - CUUR0000SAS4). The former CPI component leads the share price by 12 months and the latter one leads by 4 months. Figure 1 depicts the overall evolution of both involved indices. These two defining components provide the best fit model between August 2009 and June 2010. Both coefficients and the slope of time trend are negative. ]

So, the best-fit 2-C model for IBM(t) is as follows:

IBM(t) = 4.93MVR(t-12) – 3.51TS(t-4) - 10.39(t-2000) + 39.39

The predicted curve in Figure 2 leads the observed price by 4 months with the residual error of $5.94 for the period between July 2003 and June 2010. In other words, the price of an IBM share is completely defined by the behaviour of the two CPI components.

The model does predict the share price in the past and foresee a fall in the near future. This drop will be in line with the overall fall in the S&P 500 in 2010.

Figure 1. Evolution of the price of MEAT and IT.

Figure 2. Observed and predicted IBM share prices. The original prediction, i.e. the prediction four months before actual time, is shown by red line. Black diamonds present the original line shifted 4 months ahead to fit actual data.

Figure 3. Residual error of the model. Mean residual error is 0 with standard deviation of $5.94. The largest errors were observed in 2008.

References

Kitov, I. (2010). Deterministic mechanics of pricing. Saarbrucken, Germany, LAP Lambert Academic Publishing.

Xilinx share price

This is a funny example. According to our approach discussed in [1], the model for Xilinx (XLNX) is defined by the index of communication (CO-CUUR0000SAE2) and that of information and information processing (INF-CUUR0000SAE21). The former CPI component leads the share price by 11 months and the latter one leads by 4 months. From our past experience, the larger is the lag the more unreliable is the model. These defining components provide the best fit model, i.e. the lowermost RMS residual error, between August 2009 and June 2010. Both coefficients in the XLNX model are positive. This means that the decreasing price of communication and information (see Figure 1) forces the share price down.

So, the best-fit 2-C model for XLNX(t) is as follows:

XLNX(t) = 4.05CO(t-11) + 3.54INF(t-4) +0 .17(t-2000) + 33.25

The predicted curve in Figure 2 leads the observed price by 4 months with the residual error of $1.92 for the period between July 2003 and June 2010. In other words, the price of a XLNX share is completely defined by the behaviour of these two CPI components.

The model accurately predicts the share price in the past and foresees no significant change in the next quarter, in July through September 2010. Considering the overall fall in the S&P 500 in 2010, one should not expect any growth in this stock price at all.

Figure 1. Evolution of the price index of communication (CO) and information (INF).

Figure 2. Observed and predicted XLNX share prices.

Figure 3. Residual error of the model. Mean residual error is 0 with standard deviation of $1.92. The largest errors were observed in 2004 and 2005.

References
Kitov, I. (2010). Deterministic mechanics of pricing. Saarbrucken, Germany, LAP Lambert Academic Publishing.

Ball Corporation share price

According to [1], the model for Ball Corporation (BLL) is defined by the index of motor vehicle maintenance and repair (MVR- CUUR0000SETD) and that of communication (CO- CUUR0000SAE2). The former CPI component leads the share price by 13 months and the latter one leads by 2 months. These defining components provide the best fit model between August 2009 and June 2010.

So, the best-fit 2-C model for BLL(t) is as follows:

BLLB(t) = 2.66MVR (t-13) – 5.84(t-2) - 20.44(t-2000) + 321.46

The predicted curve in Figure 1 leads the observed price by 2 months with the residual error of $2.42 for the period between July 2003 and June 2010. In other words, the price of a BLL share is completely defined by the behaviour of the two CPI components.

The model does predict the share price in the past and foresee a significant fall in the near future. This drop will be in line with the overall fall in the S&P 500 in 2010.

Figure 1. Observed and predicted BLL share prices. Black diamonds present the original forecast shifted 2 months ahead.

References

Kitov, I. (2010). Deterministic mechanics of pricing. Saarbrucken, Germany, LAP Lambert Academic Publishing.

Schlumberger share price

According to [1], the model for Schlumberger Limited (SLB) is defined by the index of meat and meats, poultry, fish and eggs (MEAT- CUUR0000SAF112) and that of information technology (IT- CUUR0000SEEE). The former CPI component leads the share price by 2 months and the latter one leads by 6 months. Figure 1 depicts the overall evolution of both involved indices. However, both defining components provide the best fit model between August 2009 and June 2010. Both coefficients and the slope of time trend are negative.

So, the best-fit 2-C model for SLB(t) is as follows:

SLB(t) = -3.56MEAT(t-2) – 48.58IT(t-6) - 30.24(t-2000) + 1858.34

The predicted curve in Figure 2 leads the observed price by 2 months with the residual error of $6.30 for the period between July 2003 and June 2010. In other words, the price of a SLB share is completely defined by the behaviour of the two CPI components.

The model does predict the share price in the past and foresee a significant fall in the near future. This drop will be in line with the overall fall in the S&P 500 in 2010.

Figure 1. Evolution of the price of MEAT and IT.

Figure 2. Observed and predicted SLB share prices. Original prediction is shown by red line. Black diamonds present the original line shifted 2 months ahead.

Figure 3. Residual error of the model. Mean residual error is 0 with standard deviation of $6.30. The largest errors were observed in 2008.

References

Kitov, I. (2010). Deterministic mechanics of pricing. Saarbrucken, Germany, LAP Lambert Academic Publishing.

Solow on economics as a science

Robert Solow prepared a statement “Building a Science of Economics for the Real World” for the House Committee on Science and Technology, Subcommittee on Investigations and Oversight. It was presented on July 20, 2010. It is worth reading as a wonderful sample of an absolutely helpless and hopeless piece. The dry residual for science (I mean here the hard sciences) is zero. The outcome for the author criticizing a competitive “school of thought” and real economy model, a DSGE model in this case, is counterproductive.

As a matter of fact, I admire the overall discussion between mainstream economists and terminology they use to demolish rivals. Specifically, Prof. Solow used a 100% scientific term “smell” when characterized the problems and contradictions in the DSGE. It seems like a dog is sniffing around for a specific smell of other dogs …

Scientifically, if a model can not predict observations and does not pass rigorous statistical tests - it is wrong. No more, no less. In this blog, our articles, papers, and monographs, we develop, present and test only models, which do predict observations of macroeconomic variables in developed countries: real GDP, price inflation, unemployment, labor force level, S&P 500 stock market index.

We do not say that our models are 100% correct, despite they are statistically right. The logic says that correlation does not mean causality. But the absence of correlation, as all mainstream models demonstrate, 100% guarantees the absence of causality and true models. The DSGE is not excluded.

HPQ share price

According to [1], the model for Hewlett-Packard (HPQ) is defined by the index of food less beverages (FB) and that of rent of primary residency (RPR). The former CPI component leads the share price by 4 months and the latter one leads by 5 months. Figure 1 depicts the overall evolution of both involved indices. However, these two defining components provide the best fit model between August 2009 and June 2010. One coefficients is negative and one is positive together with time trend, with slope of 3.64.

So, the best-fit 2-C model for HPQ(t) is as follows:

HPG(t) = -3.20FB(t-4) +2.91RPR(t-5) + 3.64(t-2000) - 50.82

The predicted curve in Figure 2 leads the observed price by 4 months with the residual error of $2.13 for the period between July 2003 and June 2010. In other words, the price of a HPQ share is completely defined by the behaviour of the two CPI components.

The model does predict the share price in the past and foresees a fall in 2010. It will be in line with the overall fall in the S&P 500 in 2010.

Figure 1. Evolution of the price of DAIRY and TPU.

Figure 2. Observed and predicted HPQ share prices. Original prediction is shown by red line. Black diamonds present the original line shifted 4 months ahead, i.e. the model.

Figure 3. Residual error of the model. Mean residual error is 0 with standard deviation of $2.13. The largest errors were observed in 2007.

References
Kitov, I. (2010). Deterministic mechanics of pricing. Saarbrucken, Germany, LAP Lambert Academic Publishing.

MMM share price

According to [1], the model for 3M Company (MMM) is defined by the index of dairy products (DAIRY- CUUS0000SEFJ) and that of public transportation (TPU- CUUS0000SETG). The former CPI component leads the share price by 10 months and the latter one leads by 6 months. Figure 1 depicts the overall evolution of both involved indices. However, both defining components provide the best fit model between August 2009 and June 2010. Both coefficients are negative and only positive time trend with slope of 8.7 has been compensating the negative input of both CPIs .

So, the best-fit 2-C model for MMM(t) is as follows:

MMM(t) = -0.74DAIRY(t-10) – 0.54TPUP(t-6) + 8.70(t-2000) + 180.88

The predicted curve in Figure 2 leads the observed price by 6 (!) months with the residual error of $3.79 for the period between July 2003 and June 2010. In other words, the price of a MMM share is completely defined by the behaviour of the two CPI components.

The model does predict the share price in the past and foresee a significant fall in the last quarter of 2010, i.e. through December 2010. It will be in line with the overall fall in the S&P 500 in 2010.

Figure 1. Evolution of the price of DAIRY and TPU.

Figure 2. Observed and predicted MMM share prices. Original prediction is shown by red line. Black diamonds present the original line shifted 6 months ahead.

Figure 3. Residual error of the model. Mean residual error is 0 with standard deviation of $3.79. The largest errors were observed in 2005 and 2006.


References
Kitov, I. (2010). Deterministic mechanics of pricing. Saarbrucken, Germany, LAP Lambert Academic Publishing.

Predicting DeVry's share price

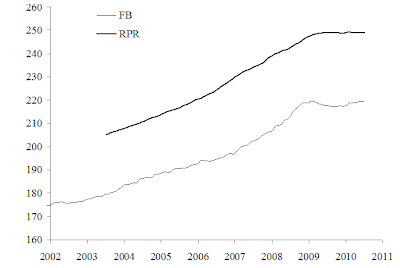
According to [1], the model for DeVry (DV) is defined by the index for the rent of primary residency (RPR-CUUS0000SEHA) and that of pets, pet products and services (PETS-CUUR0000SERB). The former CPI component leads the share price by 11 months and the latter one leads by 4 months. Figure 1 depicts the overall evolution of both involved indices. From our past experience, the larger is the lag the more unreliable is the model. However, both defining components provide the best fit model between August 2009 and June 2010. The positive influence of RPR (+7.90) is compensated by the negative input of all other terms . So, the best-fit 2-C model for DV(t) is as follows:

DV(t) =7 .90RPR(t-11) – 2.76PETS(t-4) - 35.73(t-2000) - 757.63

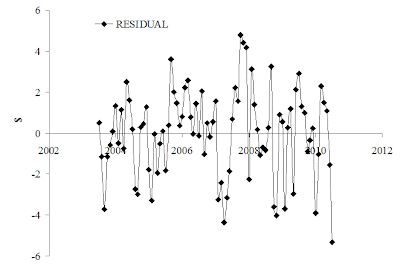
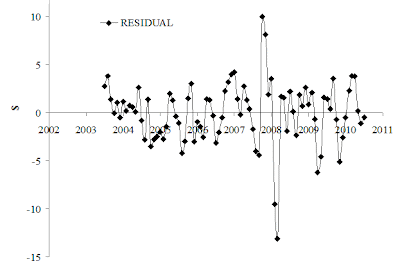
The predicted curve in Figure 2 leads the observed price by 4 months with the residual error of $3.24 for the period between July 2003 and June 2010. In other words, the price of a DV share is completely defined by the behaviour of the two CPI components.

The model does predict the share price in the past and foresees A significant fall in the next quarter, i.e. through September 2010. It will be in line with the overall fall in the S&P 500 in 2010.

Figure 1. Evolution of the price of RPR and PETS.

Figure 2. Observed and predicted DV share prices . Original predcition is shown by red line. Black diamonds present the original prediction shifted by 4 months ahead.

Figure 3. Residual error of the model. Mean residual error is 0 with standard deviation of $3.24. The largest errors were observed in 2007 and 2008.

References

Kitov, I. (2010). Deterministic mechanics of pricing. Saarbrucken, Germany, LAP Lambert Academic Publishing.

Prediction of Legg Mason share price

According to [1], the model for Legg Mason (LM) is defined by the index of food (F-CUUS0000SAF ) and that of appliances (APL-CUUR0000SEHK). The former CPI component leads the share price by 4 months and the latter one leads by 13 months. From our past experience, the larger is the lag the more unreliable is the model. However, both defining components provide the best fit model between August 2009 and June 2010. Both coefficients in the LM model are positive. This means that increasing food price forces the share price up. The fall in the index of appliances has been compensating *see Figure 1) both the increase in F and positive linear time trend in the share price, as defined by the slope of +33.024. So, the best-fit 2-C model for LM(t) is as follows:

LM(t) = 5.88F(t-4) + 8.29APL(t-13) + 33.024(t-2000) + 1425.45

The predicted curve in Figure 2 leads the observed price by 4 months with the residual error of $6.89 for the period between July 2003 and June 2010. In other words, the price of a LM share is completely defined by the behaviour of the two CPI components.

The model does predict the share price in the past and foresees no significant increase in the next quarter, in July through September 2010. Considering the overall fall in the S&P 500 in 2010, one should not expect any growth in stock prices at all.

Figure 1. Evolution of the price index of food (F) and appliances (APL).The latter has been falling since July 2009.

Figure 2. Observed and predicted LM share prices.

Figure 3. Residual error of the model. Mean residual error is 0 with standard deviation of $6.89. the largest errors were observed in 2005 and 2006.

References

Kitov, I. (2010). Deterministic mechanics of pricing. Saarbrucken, Germany, LAP Lambert Academic Publishing.

Apple share price. Revision, July 2010

Two months ago we presented a model and made a conservative prediction on AAPL share price. The model for AAPL(t), as introduced in [1], was as following

AAPL(t)= 19.03HFO(t-13) - 10.13HOS(t) + 73.86(t-1990) – 1862.5

where the index of housing furnishing and operations (HFO) leads AAPL(t) by 13 months. The index of housekeeping supplies (HOS) is synchronized with the price.

We also suggested that the price would be not growing at the same pace as in the beginning of 2010. Conditional on the decline in HOS, which was expected in April-July 2010, the share price might decline since May 2010. Figure 1 demonstrates that the actual price has been decreasing since May and this behaviour was well described by the model. One might expect that AAPL share will be falling together with S&P 500 into 2011.

Figure 1. Observed and predicted AAPL share prices.

References

Kitov, I. (2010). Deterministic mechanics of pricing. Saarbrucken, Germany, LAP Lambert Academic Publishing.

Mechanics of Inflation and Unemployment

Our monograph "Economics as Classical Mechanics" is under preparation.
We have compiled a draft version of Section 2.1 of Chapter 2 titled "Mechanics of Inflation and Unemployment". The interested reader may find Section 2.1 in pdf format here.

The contents:

2.1. Inflation, unemployment and labour force in the uSA
2.1.1. The (anti-) Phillips curve
2.1.2. Is labour force driving?
2.1.3. The model and data improvement
2.1.4. Forecasting inflation
2.1.5. Discussion

Mechanics of Gross Domestic Product

We are preparing a new monograph titled “Economics as Classical Mechanics”. This is the central part connecting two previously published monographs: “Mechanics of personal income distribution” and “Deterministic mechanics of pricing”. It is all about macroeconomics: GDP, inflation, unemployment, labor force participation rate, productivity, socialism capitalism transition. First chapater is called “Mecahnics of Gross Domestic Product”. We have prepared a draft version, which is now available here. The contents is as follows:

1.1. Real GDP per capita

1.1.1. The model

1.1.2. GDP per capita in the USA

1.1.3. Real GDP per capita Japan

1.1.4. More developed countries

1.2. Constant annual increment in real GDP per capita

1.3. Cointegration tests

1.4. Real GDP in the United States

1.5. Real GDP and S&P 500 stock market index.

We greatly appreciate any comments and criticism. The final version will likely be submitted by October 2010.

Chapter 2 (Mechanics of Inflation and Unemployment” ) is currently under initial editing and will be available for the interested readers by August 2010.

Deflation is approaching US

At last, deflation is approaching the US. A new post by Mark Thoma @ Economist's View:


How close to deflation are we?



Interstingly, five years ago we calculated that a deflationary period should start in 2012 and publsihed this forecast in 2006: Exact prediction of inflation in the USA.



Below is Figure 9 from this paper. ALl predcitions between 2006 and 2009 were almost absolutely correct. So, inflation has not come yet, but will visit the US soon.

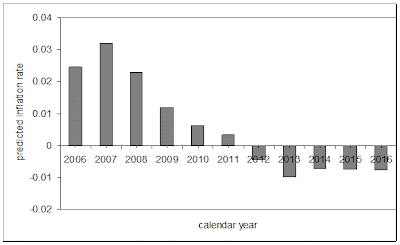
Figure 9. Predicted inflation rate for the period between 2006 and 2016.

Several months ago we presented the same graph with actual inflation readings in this blog:Sure - disinflation continues

First issue of Theoretical and Practical Research in Economic Field

The first issue of TPREF (the whole journal as a pdf file ) is available now. I am an author of one article and a co-editor.

Contents: 1	The Nexus Between Regional Growth and Technology Adoption: A Case for Club-Convergence? Stilianos Alexiadis University of Piraeus …4	7	A Survey on Labor Markets Imperfections in Mexico Using a Stochastic Frontier Juan M. Villa Inter-American Development Bank … 97
2		Can Shift to a Funded Pension System Affect National Saving? The Case of Iceland Mariangela Bonasia University of Naples Oreste Napolitano University of Naples … 12
3		Global Supply Chains and the Great Trade Collapse: Guilty or Casualty? Hubert Escaith World Trade Organization … 27
4		Some Empirical Evidence of the Euro Area Monetary Policy Antonio Forte University of Bari … 42
5		Modeling Share Prices of Banks and Bankrupts Ivan O. Kitov Institute for the Geospheres‟ Dynamics, Russian Academy of Sciences … 59
6		Infrastructures and Economic Performance: A Critical Comparison Across Four Approaches Gianpiero Torrisi Newcastle University … 86

Unemployment In Germany

Approximately a year ago we predicted the rate of unemployment, UE, in Germany at the level of 11% in 2011. This prediction was obtained from the following model linking unemployment and labor force, LF:

UE(t) = 3.2dLF(t-5)/LF(t-5) + 0.08 (1),

where the change in labor force leads the unemployment by 5 (!) years. A comprehensive discussion of the model and data, as retrieved from OECD database, is given in [1].

For this study, we borrowed unemployment estimates from the DEStatis (Federal Statistics Office). They are slightly different from those provided by the OECD, and are issued at a monthly rate.

This is time to revise the prediction and estimates. Figure 1 presents the measured and predicted unemployment rate for the period between 2002 and 2012. All in all, both curves are very close, except the most recent period.

Because of the discrepancy, we have checked the DEStatis for corroborative data on labor force and found new estimates for 2006 through 2009, which are related to national concept. When (1) is applied, one obtains the open triangle curve with the new estimates.

We still consider the level of 11% in 2011 as a reliable estimate. From 2001 to 2009 relationship (1) worked well with the estimates of labor force from the OECD.

However, there is a possibility that the new estimates of labor force are not too bad, and actual unemployment in 2011 will not be above 10%. In 2010 the rate will reach the level between 8% and 9%.

This is a good example that one can predict the future evolution of macroeconomic variables, but the past is unpredcitable. It is a common feature when statistical agencies revise their past estimates.

Figure 2. Observed and predicted rate of unemployment in Germany.

Second half of 2010 - second wave of crisis

As discussed in our working paper, there exists a trade-off between the growth rate of real GDP, G(t), and the S&P 500 returns, R(t). The predicted returns, R_p(t), can be obtained from the following relationship:

R_p(t) = 0.0062dlnG(t) - 0.01,

where G(t) is represented by (six month moving average) MA(6) of the (annualized) growth rate during six previous months or two quarters, because only quarterly readings of real GDP are available.

Figure 1 displays the observed S&P 500 returns and those obtained using real GDP, as presented by the US Bureau of Economic Analysis. The observed returns are MA(12) of the monthly returns. The period after 1996 is relatively well predicted including the increase in 2003. Therefore, it is reasonable to assume that G(t) can be used for modeling of the S&P 500 index and returns. Reciprocally, current S&P 500 may be used for the estimation of GDP.

In the previous article, we have predicted the future of S&P 500 and its returns. Now we invert the predicted figures and calculate real GDP for the same period. The bets-fit GDP figures are obtained from the cumulative curves shown in Figure 2. Our estimates from 2009Q3 to 2010Q4 are shown by red circles and red diamonds. The estimated GDP growth rates are as follows:

2009Q3: 3% (2.2%)
2009Q4: 7% (5.6%)
2010Q1: 6% (2.6%)
2010Q2: +2%
2010Q3: -2%
2010Q4: -3%

In the brackets, the current estimates of the growth rate of real GDP are given, which will be all revised in July 2010. So, our model shows that GDP was slightly underestimated in the second half of 2009 and heavily underestimated in the first quarter of 2010. In 2010Q2 the growth will slow down and then a period of GDP contraction will start. Some people call it the second wave of crisis. This is what our model foresees.

Figure 1. Observed and predicted S&P 500 returns 1985 to 2011.The future S&P 500 returns are converted into GDP growth rates. Corresponding re-estimates of the returns are shown by red diamonds.

Figure 2. Cumulative observed and predicted S&P 500 returns. Red diamonds represent GDP figures which fit the predicted S&P 500 returns.

S&P 500 in July 2010

We continue tracking the evolution of the S&P 500 and our prediction made in the beginning of 2009 for the next six years. Since March 2009, the prediction fits the observed S&P 500 with minor deviations likely related to the emotion component of the stock market. However, the trend and its turn in May 2010 were forecasted precisely. All in all, fifteen months in a row we are right and do not see any source which may disturb our prediction for the period between June 2010 and 2014. The prediction was documented in a working paper (S&P 500 returns revisited) and several posts .

The original model links the S&P 500 annual returns, R_p(t), to the number of nine-year-olds, N₉. To obtain a prediction we use the number of three-year-olds, N₃, as a proxy to N₉ at a six-year horizon:

R_p(t+6) = 100dlnN₃(t) - 0.23

where R_p(t+6)is the S&P 500 return at a six-year horizon. Because of the properties of the N₃ distribution one can replace it with linear trends for the period between 2008 and 2011, as Figure 1 shows. The model shown in Figure 1 predicts that the S&P 500 stock market index will be gradually decreasing at an average rate of 37 points per month. (Correction from the previous post where 46 points per months was used by mistake.) In June, actual closing level was 1030 (-60 relative to May 2010). This level is about 90 points below that predicted in Figure 1. This is the continuation of the May’s panic. Such dynamic "overshoot" in the beginning of a new trend is a common feature.

Figure 1. Observed S&P 500 monthly close level and the trend predicted from the number of nine-year-olds. The slope is of -37 points per month. The same but positive slope was observed between February 2009 and April 2010.

The deviation from the new trend is a big one and one can expect the end of panic in July/August 2010. This is a nice feature of the trend. Any deviation, whatever amplitude it has, must return to the trend. So, by the past experience we may judge that 90 points should be compensated quickly. This means that the level of S&P 500 should not change much in July and August 2010. We would expect the close level between 1020 and 1050 in July 2010.

Then, the index will continue gradual decrease into 2011. Figure 2 demonstrates that the S&P 500 annual return will sink below zero in the third-fourth quarter of 2010.

Figure 2. Observed and predicted S&P 500 returns.