Predicting DeVry's share price

According to [1], the model for DeVry (DV) is defined by the index for the rent of primary residency (RPR-CUUS0000SEHA) and that of pets, pet products and services (PETS-CUUR0000SERB). The former CPI component leads the share price by 11 months and the latter one leads by 4 months. Figure 1 depicts the overall evolution of both involved indices. From our past experience, the larger is the lag the more unreliable is the model. However, both defining components provide the best fit model between August 2009 and June 2010. The positive influence of RPR (+7.90) is compensated by the negative input of all other terms . So, the best-fit 2-C model for DV(t) is as follows:

DV(t) =7 .90RPR(t-11) – 2.76PETS(t-4) - 35.73(t-2000) - 757.63

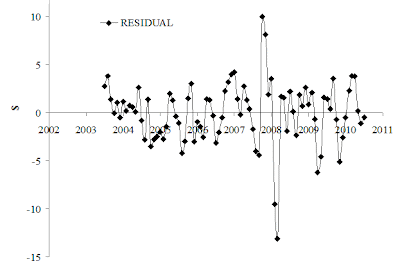
The predicted curve in Figure 2 leads the observed price by 4 months with the residual error of $3.24 for the period between July 2003 and June 2010. In other words, the price of a DV share is completely defined by the behaviour of the two CPI components.

The model does predict the share price in the past and foresees A significant fall in the next quarter, i.e. through September 2010. It will be in line with the overall fall in the S&P 500 in 2010.

Figure 1. Evolution of the price of RPR and PETS.

Figure 2. Observed and predicted DV share prices . Original predcition is shown by red line. Black diamonds present the original prediction shifted by 4 months ahead.

Figure 3. Residual error of the model. Mean residual error is 0 with standard deviation of $3.24. The largest errors were observed in 2007 and 2008.

References

Kitov, I. (2010). Deterministic mechanics of pricing. Saarbrucken, Germany, LAP Lambert Academic Publishing.